Toroidal coordinates: Difference between revisions

From FusionWiki
Jump to navigation Jump to search
 
(64 intermediate revisions by 35 users not shown)
Line 10: Line 10:
== Cylindrical coordinates ==
== Cylindrical coordinates ==


(''R'', ''φ'', ''Z''), where  
<math>(R, \phi, Z)</math>, where  
<ref>[[:Wikipedia:Cylindrical coordinate system]]</ref>
<ref>[[:Wikipedia:Cylindrical coordinate system]]</ref>
* ''R<sup>2</sup> = X<sup>2</sup> + Y<sup>2</sup>'', and  
* <math>R^2 = X^2 + Y^2</math>, and  
* tan ''&phi;'' = ''Y/X''.  
* <math>\tan \phi = Y/X</math>.  


''&phi;'' is called the ''toroidal angle'' (and not the ''cylindrical'' angle, at least not in the context of magnetic confinement).
<math>\phi</math> is called the ''toroidal angle'' (and not the ''cylindrical'' angle, at least not in the context of magnetic confinement).


Cylindrical symmetry (symmetry under rotation over &phi;) is referred to as ''axisymmetry''.
Cylindrical symmetry (symmetry under rotation over <math>\phi</math>) is referred to as ''[[axisymmetry]]''.


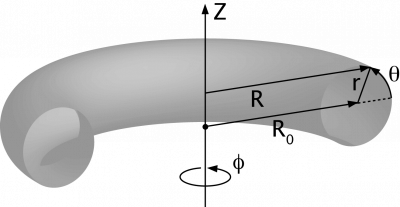
== Simple toroidal coordinates ==
== Simple toroidal coordinates ==


(''r'', ''&phi;'', ''&theta;''), where  
<math>(r, \phi, \theta)</math>, where  
* ''R'' = ''R<sub>0</sub>'' + ''r'' cos ''&theta;'', and
* <math>R = R_0 + r \cos \theta</math>, and
* ''Z'' = ''r'' sin ''&theta;''
* <math>Z = r \sin \theta</math>
''R<sub>0</sub>'' corresponds to the torus axis and is called the ''major radius'', while ''r'' is called the ''minor radius'', and ''&theta;'' the ''poloidal angle''.
<math>R_0</math> corresponds to the torus axis and is called the ''major radius'', while <math>0 \le r \le a</math> is called the ''minor radius'', and ''&theta;'' the ''poloidal angle''.
The ratio <math>R_0/a</math> is called the ''aspect ratio'' of the torus.


In order to adapt this simple system better to the [[Flux surface|magnetic surfaces]] of an axisymmetric [[MHD equilibrium]], it may be enhanced by
In order to adapt this simple system better to the [[Flux surface|magnetic surfaces]] of an axisymmetric [[MHD equilibrium]], it may be enhanced by
<ref>[http://link.aip.org/link/?PHPAEN/5/973/1 R.L. Miller et al, ''Noncircular, finite aspect ratio, local equilibrium model'', Phys. Plasmas '''5''' (1998) 973]</ref>
<ref>R.L. Miller et al, ''Noncircular, finite aspect ratio, local equilibrium model'', [[doi:10.1063/1.872666|Phys. Plasmas '''5''' (1998) 973]]</ref>
* letting ''R<sub>0</sub>'' depend on ''r'' (to account for the [[Shafranov shift]] of flux surfaces) <ref>R.D. Hazeltine, J.D. Meiss, ''Plasma confinement'', Courier Dover Publications (2003) ISBN 0486432424</ref>
* letting <math>R_0/a</math> depend on <math>r</math> (to account for the [[Shafranov shift]] of flux surfaces) <ref>R.D. Hazeltine, J.D. Meiss, ''Plasma confinement'', Courier Dover Publications (2003) {{ISBN|0486432424}}</ref>
* adding ellipticity (''&epsilon;''), triangularity (''&kappa;''), etc. (to account for non-circular flux surface cross sections)
* adding [[ellipticity]] (<math>\kappa</math>), [[triangularity]] (<math>\delta</math>), and squareness (<math>\zeta</math>) to account for non-circular flux surface cross sections. A popular simple expression for shaped flux surfaces is: <ref> R.L. Miller, M.S. Chu, J.M. Greene, Y.R. Lin-Liu and R.E. Waltz, ''Noncircular, finite aspect ratio, local equilibrium model'', [[doi:10.1063/1.872666|Phys. Plasmas '''5''' (1998) 973]]</ref>
 
:<math>R(r,\theta) = R_0(r) + r \cos(\theta + \arcsin \delta \sin \theta)</math>
:<math>Z(r,\theta) = Z_0(r) + \kappa(r) r \sin(\theta + \zeta \sin 2 \theta) </math>
 
Warning: there are varying conventions for the directions of <math>\theta</math> and <math>\phi</math>. Which convention is used can depend on the local facility, the software being used, or other context. To help reduce confusion, the different tokamak coordinate conventions have been described and codified in the COCOS system.<ref>O. Sauter and S.Yu. Medvedev, ''Tokamak coordinate conventions: COCOS'', [[doi:10.1016/j.cpc.2012.09.010|Computer Physics Communications '''184''', (2013) 293-302]]</ref>


== Toroidal coordinates ==
== Toroidal coordinates ==


(''&zeta;'', ''&eta;'', ''&phi;''), where
<math>(\zeta, \eta, \phi)</math>, where
<ref>Morse and Feshbach, ''Methods of theoretical physics'', McGraw-Hill, New York, 1953 ISBN 007043316X</ref>
<ref>Morse and Feshbach, ''Methods of theoretical physics'', McGraw-Hill, New York, 1953 {{ISBN|007043316X}}</ref>
<ref>[[:Wikipedia:Toroidal coordinates]]</ref>
<ref>[[:Wikipedia:Toroidal coordinates]]</ref>


Line 41: Line 47:
:<math>Z = R_p \frac{\sin \eta}{\cosh \zeta - \cos \eta}</math>
:<math>Z = R_p \frac{\sin \eta}{\cosh \zeta - \cos \eta}</math>


where ''R<sub>p</sub>'' is the pole of the coordinate system.  
where <math>R_p</math> is the pole of the coordinate system.  
Surfaces of constant ''&zeta;'' are tori with major radii ''R = R<sub>p</sub>''/tanh ''&zeta;'' and minor radii ''r = R<sub>p</sub>''/sinh ''&zeta;''.  
Surfaces of constant <math>\zeta</math> are tori with major radii <math>R = R_p/\tanh \zeta</math> and minor radii <math>r = R_p/\sinh \zeta</math>.  
At ''R = R<sub>p</sub>'', ''&zeta;'' = &infin;, while at infinity and at ''R = 0, &zeta; = 0''.  
At <math>R = R_p</math>, <math>\zeta = \infty</math>, while at infinity and at <math>R = 0, \zeta = 0</math>.  
The coordinate ''&eta;'' is a poloidal angle and runs from 0 to 2&pi;.  
The coordinate <math>\eta</math> is a poloidal angle and runs from 0 to <math>2\pi</math>.  
This system is orthogonal.
This system is orthogonal.


The Laplace equation separates in this system of coordinates, thus allowing an expansion of the vacuum magnetic field in toroidal harmonics.
The Laplace equation separates in this system of coordinates, thus allowing an expansion of the vacuum magnetic field in toroidal harmonics.
<ref>F. Alladio, F. Chrisanti, ''Analysis of MHD equilibria by toroidal multipolar expansions'', Nucl. Fusion '''26''' (1986) 1143</ref>
<ref>F. Alladio, F. Crisanti, ''Analysis of MHD equilibria by toroidal multipolar expansions'', [[doi:10.1088/0029-5515/26/9/002|Nucl. Fusion '''26''' (1986) 1143]]</ref>
<ref>[http://dx.doi.org/10.1016/0010-4655(94)90112-0 B.Ph. van Milligen and A. Lopez Fraguas, ''Expansion of vacuum magnetic fields in toroidal harmonics'', Computer Physics Communications '''81''', Issues 1-2 (1994) 74-90]</ref>
<ref>B.Ph. van Milligen and A. Lopez Fraguas, ''Expansion of vacuum magnetic fields in toroidal harmonics'', [[doi:10.1016/0010-4655(94)90112-0|Computer Physics Communications '''81''', Issues 1-2 (1994) 74-90]]</ref>
 
== General curvilinear coordinates ==
Here we briefly review the basic definitions of a general [[:Wikipedia:Curvilinear coordinates | curvilinear coordinate system]] for later convenience when discussing toroidal flux coordinates and magnetic coordinates.
 
=== Function coordinates and basis vector ===
Given the spatial dependence of a coordinate set <math>(\psi(\mathbf{x}),\theta(\mathbf{x}),\phi(\mathbf{x}))</math>
we can calculate the contravariant basis vectors
:<math>
\mathbf{e}^i = \{\nabla\psi, \nabla\theta, \nabla\phi\}
</math>
and the dual covariant basis defined as
:<math>
\mathbf{e}_i= \frac{\partial\mathbf{x}}{\partial{u^i}}
\to
\mathbf{e}_i\cdot\mathbf{e}^j
= \delta_{i}^{j} \to \mathbf{e}_i
= \frac{\mathbf{e}^j\times\mathbf{e}^k}{|\mathbf{e}^i\cdot\mathbf{e}^j\times\mathbf{e}^k|}
= \sqrt{g}\;\mathbf{e}^j\times\mathbf{e}^k ~,
</math>
where <math>(i,j,k)</math> are cyclic permutations of <math>(1,2,3)</math> and we have used the notation <math>(u^1, u^2, u^3) = (\psi,\theta,\phi)</math>. The Jacobian <math>\sqrt{g}</math> is defined below.
 
Any vector field <math>\mathbf{B}</math> can be represented as
:<math>
\mathbf{B}
= (\mathbf{B}\cdot\mathbf{e}^i)\mathbf{e}_i
= B^i\mathbf{e}_i
</math>
or
:<math>
\mathbf{B}
= (\mathbf{B}\cdot\mathbf{e}_i)\mathbf{e}^i
= B_i\mathbf{e}^i ~.
</math>
In particular any basis vector <math>\mathbf{e}_i = (\mathbf{e}_i\cdot\mathbf{e}_j)\mathbf{e}^j</math>. The metric tensor is defined as
:<math>
g_{ij}
= \mathbf{e}_i\cdot\mathbf{e}_j
\; ; \;
g^{ij}
= \mathbf{e}^i\cdot\mathbf{e}^j
\; ; \;
g^j_i 
= \mathbf{e}_i\cdot\mathbf{e}^j = \delta_i^j ~.
</math>
 
=== Jacobian ===
The Jacobian of the coordinate transformation <math>\mathbf{x}(\psi, \theta, \phi)</math> is defined as
:<math>
J = \det\left(\frac{\partial(x,y,z)}{\partial(\psi,\theta,\phi)}\right) = \frac{\partial\mathbf{x}}{\partial{\psi}}\cdot\frac{\partial\mathbf{x}}{\partial{\theta}} \times \frac{\partial\mathbf{x}}{\partial{\phi}}
</math>
and that of the inverse transformation
:<math>
J^{-1} = \det\left(\frac{\partial(\psi,\theta,\phi)}{\partial(x,y,z)}\right) = \nabla{\psi}\cdot\nabla{\theta} \times \nabla{\phi}
</math>
It can be seen that <ref name='Dhaeseleer'></ref> <math>g \equiv \det(g_{ij}) = J^2 \Rightarrow J = \sqrt{g}</math>
 
== Flux coordinates ==
A flux coordinate set is one that includes a [[Flux surface|flux surface]] label as a coordinate. A flux surface label is a function that is constant and single valued on each flux surface. In our naming of the general curvilinear coordinates we have already adopted the usual flux coordinate convention for toroidal equilibrium with nested flux surfaces with <math>\psi</math> being the flux surface label and <math>\theta, \phi</math> are <math>2\pi</math>-periodic poloidal and toroidal-like angles.
 
Different flux surface labels can be chosen like toroidal or poloidal magnetic fluxes or the volume contained within the flux surface. By single valued we mean to ensure that any flux label <math>\psi_1 = f(\psi_2)</math> is a monotonous function of any other flux label  <math>\psi_2</math>, so that the function  <math>f</math> is invertible at least in a volume containing the region of interest.
=== Flux Surface Average ===
The flux surface average of a function <math>\Phi</math> is defined as the limit
:<math>
\langle\Phi\rangle = \lim_{\delta V \to 0}\frac{1}{\delta V}\int_{\delta V} \Phi\; dV
</math>
where <math>\delta V</math> is the volume confined between two flux surfaces. It is therefore a ''volume average'' over an infinitesimal spatial region rather than a surface average.
 
Introducing the differential volume element <math>dV = \sqrt{g} d\psi d\theta d\phi</math>
:<math>
\langle\Phi\rangle
= \lim_{\delta V \to 0} \frac{1}{\delta V}\int_{\delta V} \Phi\; \sqrt{g} d\psi d\theta d\phi
= \frac{d\psi}{d V}\int_0^{2\pi}\int_0^{2\pi}\Phi\; \sqrt{g} d\theta d\phi
</math>
or, noting that <math>\langle 1\rangle = 1</math>, we have <math>\frac{dV}{d\psi} = \int_0^{2\pi}\int_0^{2\pi} \sqrt{g} d\theta d\phi</math> and
we get to a more practical form of the Flux Surface Average
:<math>
\langle\Phi\rangle
= \frac{\int_0^{2\pi}\int_0^{2\pi}\Phi\; \sqrt{g} d\theta d\phi}
{\int_0^{2\pi}\int_0^{2\pi} \sqrt{g} d\theta d\phi}
</math>
 
Note that <math>dS = |\nabla\psi|\sqrt{g}d\theta d\phi</math>, so the FSA is a surface integral ''weighted by'' <math>|\nabla V|^{-1}</math> :
:<math>
\langle\Phi\rangle
= \frac{d\psi}{d V}\int_0^{2\pi}\int_0^{2\pi}\Phi\; \sqrt{g} d\theta d\phi
= \frac{d\psi}{d V}\int_{S(\psi)}\frac{\Phi}{|\nabla\psi|}\; dS
= \int_{S(\psi)}\frac{\Phi}{|\nabla V|}\; dS
</math>
 
Applying Gauss' theorem to the definition of FSA we get to the identity
:<math>
\langle\nabla\cdot\Gamma\rangle
= \lim_{\delta V \to 0}\frac{1}{\delta V}\int_{\delta V} \nabla\cdot\Gamma\; dV
= \lim_{\delta V \to 0}\frac{1}{\delta V}\int_{S(\delta V)} \Gamma\cdot \frac{\nabla V}{|\nabla V|}dS
= \lim_{\delta V \to 0}\frac{1}{\delta V}\int_0^{2\pi} \int_0^{2\pi} \Gamma\cdot \nabla V\; \sqrt{g} d\theta d\phi = \frac{d}{dV}\langle\Gamma\cdot\nabla V\rangle~.
</math>
 
==== Useful properties of FSA ====
Some useful properties of the FSA are
 
*<math> \langle\nabla\cdot\Gamma\rangle = \frac{d}{dV}\langle\Gamma\cdot\nabla V\rangle  = \frac{1}{V'}\frac{d}{d\psi}V'\langle\Gamma\cdot\nabla \psi\rangle</math>
*<math> \int_{V}\nabla\cdot\Gamma\; dV =  \langle\Gamma\cdot\nabla V\rangle = V'\langle\Gamma\cdot\nabla \psi\rangle</math>
*<math> \langle \sqrt{g}^{-1}\rangle = \frac{4\pi^2}{V'}
</math>
*<math> \langle \mathbf{B}\cdot\nabla f \rangle = 0~,\qquad \forall~ \mathrm{single~valued~} f(\mathbf{x}), ~ \mathrm{if}~ \nabla\cdot\mathbf{B} = 0 ~\mathrm{and}~ \nabla V\cdot\mathbf{B} = 0 </math>
*<math> \langle \mathbf{B}\cdot\nabla \theta\rangle =2\pi\frac{d\Psi_{pol}}{dV} \qquad (\mathrm{Note:}~ \theta(\mathbf{x})~\mathrm{is~not~single~valued})
</math>
*<math> \langle \mathbf{B}\cdot\nabla \phi\rangle =2\pi\frac{d\Psi_{tor}}{dV} \qquad (\mathrm{Note:}~ \phi(\mathbf{x})~\mathrm{is~not~single~valued})
</math>
where <math>V' = \frac{dV}{d\psi}</math>.
 
=== Magnetic field representation in flux coordinates ===
 
==== Contravariant From ====
Any [[:Wikipedia: solenoidal vector field| solenoidal vector field]] <math>\mathbf{B}</math>  can be written as
<math> \mathbf{B} = \nabla\alpha\times\nabla\nu </math>
called its Clebsch representation. For a magnetic field with flux surfaces <math>(\psi = \mathrm{const}\; , \; \nabla\psi\cdot\mathbf{B} = 0)</math> we can choose, say, <math>\alpha</math> to be the flux surface label <math>\psi</math>
:<math>
\mathbf{B} = \nabla\psi\times\nabla\nu
</math>
Field lines are then given as the intersection of the constant-<math>\psi</math> and constant-<math>\nu</math> surfaces. This form provides a general expression for <math>\mathbf{B}</math> in terms of the covariant basis vectors of a flux coordinate system
:<math>
\mathbf{B} = \frac{\partial\nu}{\partial\theta}\nabla\psi\times\nabla\theta + \frac{\partial\nu}{\partial\phi}\nabla\psi\times\nabla\phi =  \frac{1}{\sqrt{g}}\frac{\partial\nu}{\partial\theta}\mathbf{e}_\phi -\frac{1}{\sqrt{g}}\frac{\partial\nu}{\partial\phi}\mathbf{e}_\theta = B^\phi\mathbf{e}_\phi + B^\theta\mathbf{e}_\theta~.
</math>
in terms of the function <math>\nu</math>, sometimes referred to as the magnetic field's ''stream function''.
 
It is worthwhile to note that the Clebsch form of <math> \mathbf{B} </math> corresponds to a [[:Wikipedia: Magnetic potential|magnetic vector potential]]
<math> \mathbf{A} = \nu\nabla\psi </math> (or <math> \mathbf{A} = \psi\nabla\nu </math> as they differ only by the Gauge transformation <math> \mathbf{A} \to \mathbf{A} - \nabla (\psi\nu)</math>).
 
The general form of the stream function is
:<math>
\nu(\psi,\theta,\phi)
= \frac{1}{2\pi}(\dot{\Psi}_{tor}\theta
- \dot{\Psi}_{pol}\phi)
+ \tilde{\nu}(\psi,\theta,\phi)
</math>
where <math>\tilde{\nu}</math> is a differentiable function periodic in the two angles. This general form can be derived by using the fact that  <math> \mathbf{B}</math> is a physical function (hence singe-valued). The specific form for the coefficients in front of the secular terms (i.e. the non-periodic terms) can be obtained from the [[Useful properties of FSA|FSA properties ]].
 
==== Covariant Form ====
 
If we consider an equilibrium magnetic field such that <math> \mathbf{j}\times\mathbf{B} \propto \nabla\psi</math>, then both <math> \mathbf{B}\cdot\nabla\psi = 0</math> and <math> \nabla\times\mathbf{B}\cdot\nabla\psi = 0</math> and the magnetic field can be written as
:<math>
\mathbf{B} = \beta\nabla\psi + \nabla\chi
</math>
where <math>\chi</math> is identified as the magnetic ''scalar'' potential. Its general form is
:<math>
\chi(\psi, \theta, \phi) = \frac{I_{tor}}{2\pi}\theta + \frac{I_{pol}^d}{2\pi}\phi + \tilde\chi(\psi, \theta, \phi)
</math>
The functional dependence on the angular variables is again motivated by the single-valuedness of the magnetic field. The particular form of the coefficients can be obtained noting that
:<math>
\int_S \mu_0\mathbf{j}\cdot d\mathbf{S}
= \int_{\partial S}\mathbf{B}\cdot d\mathbf{l}
= \oint(\beta\nabla\psi + \nabla\chi)\cdot d\mathbf{l}
= \oint(\beta d\psi + d\chi)
</math>
and choosing an integration circuit contained within a flux surface <math>(d\psi = 0)</math>. Then we get
:<math>
\int_S \mu_0\mathbf{j}\cdot d\mathbf{S}
= \Delta \chi = \frac{I_{tor}}{2\pi}\Delta\theta + \frac{I_{pol}^d}{2\pi}\Delta\phi~.
</math>
 
[[Image:CurrentIntegrationCirtuits.png|thumb|right|alt=Sample integration circuits for the definitions of currents.|Sample integration circuits for the current definitions.]]
 
If we now chose a ''toroidal'' circuit <math>(\Delta\theta = 0, \Delta\phi = 2\pi)</math> we get
:<math>
I_{pol}^d = \int_S \mu_0\mathbf{j}\cdot d\mathbf{S}\; ; ~\mathrm{with}~ \partial S ~\mathrm{such~that}~ (\Delta\theta = 0, \Delta\phi = 2\pi)~.
</math>
here the superscript <math>d</math> is meant to indicate the flux is computed through a disc limited by the integration line, as opposed to the ribbon limited by the integration line on one side and the magnetic axis on the other that was used for the definition of poloidal magnetic flux <math>\Psi_{pol}</math> above these lines. 
Similarly
:<math>
I_{tor} = \int_S \mu_0\mathbf{j}\cdot d\mathbf{S}\; ; ~\mathrm{with}~ \partial S ~\mathrm{such~that}~ (\Delta\theta = 2\pi, \Delta\phi = 0)~.
</math>


== Magnetic coordinates ==
== Magnetic coordinates ==
 
Magnetic coordinates are a particular type of [[flux coordinates]] in which the magnetic field lines are straight lines. Magnetic coordinates adapt to the magnetic field, and therefore to the [[MHD equilibrium]] (also see [[Flux surface]]).  
Magnetic coordinates adapt to the magnetic field, and therefore to the [[MHD equilibrium]] (also see [[Flux surface]]).  
Magnetic coordinates simplify the description of the magnetic field.  
Magnetic coordinates simplify the description of the magnetic field.  
In 3 dimensions (not assuming axisymmetry), the most commonly used coordinate systems are:
In 3 dimensions (not assuming axisymmetry), the most commonly used coordinate systems are:
<ref name='Dhaeseleer'>W.D. D'haeseleer, ''Flux coordinates and magnetic field structure: a guide to a fundamental tool of plasma theory'', Springer series in computational physics, Springer-Verlag (1991) ISBN 3540524193</ref>
<ref name='Dhaeseleer'>W.D. D'haeseleer, ''Flux coordinates and magnetic field structure: a guide to a fundamental tool of plasma theory'', Springer series in computational physics, Springer-Verlag (1991) {{ISBN|3540524193}}</ref>
* Hamada coordinates. <ref>S. Hamada, Nucl. Fusion '''2''' (1962) 23</ref><ref>[http://dx.doi.org/10.1063/1.1706651 J.M. Greene and J.L Johnson, ''Stability Criterion for Arbitrary Hydromagnetic Equilibria'', Phys. Fluids '''5''' (1962) 510]</ref> In these coordinates, both the field lines and current lines corresponding to the [[MHD equilibrium]] are straight.  
* [[Hamada coordinates]]. <ref>S. Hamada, [[doi:10.1088/0029-5515/2/1-2/005|Nucl. Fusion '''2''' (1962) 23]]</ref><ref>J.M. Greene and J.L Johnson, ''Stability Criterion for Arbitrary Hydromagnetic Equilibria'', [[doi:10.1063/1.1706651|Phys. Fluids '''5''' (1962) 510]]</ref> In these coordinates, both the field lines and current lines corresponding to the [[MHD equilibrium]] are straight.
* Boozer coordinates. <ref>[http://dx.doi.org/10.1063/1.863297 A.H. Boozer, ''Plasma equilibrium with rational magnetic surfaces'', Phys. Fluids '''24''' (1981) 1999]</ref><ref>[http://dx.doi.org/10.1063/1.863765 A.H. Boozer, ''Establishment of magnetic coordinates for a given magnetic field'', Phys. Fluids '''25''' (1982) 520]</ref> In these coordinates, the field lines corresponding to the [[MHD equilibrium]] are straight.
* [[Boozer coordinates]]. <ref>A.H. Boozer, ''Plasma equilibrium with rational magnetic surfaces'', [[doi:10.1063/1.863297|Phys. Fluids '''24''' (1981) 1999]]</ref><ref>A.H. Boozer, ''Establishment of magnetic coordinates for a given magnetic field'', [[doi:10.1063/1.863765|Phys. Fluids '''25''' (1982) 520]]</ref> In these coordinates, the field lines corresponding to the [[MHD equilibrium]] are straight and so are the ''diamagnetic lines '', i.e. the integral lines of <math>\nabla\psi\times\mathbf{B}</math>.
 
These two coordinate systems are related.
These two coordinate systems are related.
<ref>K. Miyamoto, ''Controlled fusion and plasma physics'', Vol. 21 of Series in
<ref>K. Miyamoto, ''Controlled fusion and plasma physics'', Vol. 21 of Series in
Plasma Physics, CRC Press (2007) ISBN 1584887095</ref>
Plasma Physics, CRC Press (2007) {{ISBN|1584887095}}</ref>


== References ==
== References ==
<references />
<references />

Latest revision as of 12:13, 12 December 2023

A simple toroidal coordinate system

Coordinate systems used in toroidal systems:

Cartesian coordinates

(X, Y, Z) [1]

Cylindrical coordinates

, where [2]

  • , and
  • .

is called the toroidal angle (and not the cylindrical angle, at least not in the context of magnetic confinement).

Cylindrical symmetry (symmetry under rotation over ) is referred to as axisymmetry.

Simple toroidal coordinates

, where

  • , and

corresponds to the torus axis and is called the major radius, while is called the minor radius, and θ the poloidal angle. The ratio is called the aspect ratio of the torus.

In order to adapt this simple system better to the magnetic surfaces of an axisymmetric MHD equilibrium, it may be enhanced by [3]

  • letting depend on (to account for the Shafranov shift of flux surfaces) [4]
  • adding ellipticity (), triangularity (), and squareness () to account for non-circular flux surface cross sections. A popular simple expression for shaped flux surfaces is: [5]

Warning: there are varying conventions for the directions of and . Which convention is used can depend on the local facility, the software being used, or other context. To help reduce confusion, the different tokamak coordinate conventions have been described and codified in the COCOS system.[6]

Toroidal coordinates

, where [7] [8]

where is the pole of the coordinate system. Surfaces of constant are tori with major radii and minor radii . At , , while at infinity and at . The coordinate is a poloidal angle and runs from 0 to . This system is orthogonal.

The Laplace equation separates in this system of coordinates, thus allowing an expansion of the vacuum magnetic field in toroidal harmonics. [9] [10]

Magnetic coordinates

Magnetic coordinates are a particular type of flux coordinates in which the magnetic field lines are straight lines. Magnetic coordinates adapt to the magnetic field, and therefore to the MHD equilibrium (also see Flux surface). Magnetic coordinates simplify the description of the magnetic field. In 3 dimensions (not assuming axisymmetry), the most commonly used coordinate systems are: [11]

These two coordinate systems are related. [16]

References

  1. ↑ Wikipedia:Cartesian coordinate system
  2. ↑ Wikipedia:Cylindrical coordinate system
  3. ↑ R.L. Miller et al, Noncircular, finite aspect ratio, local equilibrium model, Phys. Plasmas 5 (1998) 973
  4. ↑ R.D. Hazeltine, J.D. Meiss, Plasma confinement, Courier Dover Publications (2003) ISBN 0486432424
  5. ↑ R.L. Miller, M.S. Chu, J.M. Greene, Y.R. Lin-Liu and R.E. Waltz, Noncircular, finite aspect ratio, local equilibrium model, Phys. Plasmas 5 (1998) 973
  6. ↑ O. Sauter and S.Yu. Medvedev, Tokamak coordinate conventions: COCOS, Computer Physics Communications 184, (2013) 293-302
  7. ↑ Morse and Feshbach, Methods of theoretical physics, McGraw-Hill, New York, 1953 ISBN 007043316X
  8. ↑ Wikipedia:Toroidal coordinates
  9. ↑ F. Alladio, F. Crisanti, Analysis of MHD equilibria by toroidal multipolar expansions, Nucl. Fusion 26 (1986) 1143
  10. ↑ B.Ph. van Milligen and A. Lopez Fraguas, Expansion of vacuum magnetic fields in toroidal harmonics, Computer Physics Communications 81, Issues 1-2 (1994) 74-90
  11. ↑ W.D. D'haeseleer, Flux coordinates and magnetic field structure: a guide to a fundamental tool of plasma theory, Springer series in computational physics, Springer-Verlag (1991) ISBN 3540524193
  12. ↑ S. Hamada, Nucl. Fusion 2 (1962) 23
  13. ↑ J.M. Greene and J.L Johnson, Stability Criterion for Arbitrary Hydromagnetic Equilibria, Phys. Fluids 5 (1962) 510
  14. ↑ A.H. Boozer, Plasma equilibrium with rational magnetic surfaces, Phys. Fluids 24 (1981) 1999
  15. ↑ A.H. Boozer, Establishment of magnetic coordinates for a given magnetic field, Phys. Fluids 25 (1982) 520
  16. ↑ K. Miyamoto, Controlled fusion and plasma physics, Vol. 21 of Series in Plasma Physics, CRC Press (2007) ISBN 1584887095
Retrieved from "http://wiki.fusenet.eu/fusionwiki/index.php?title=Toroidal_coordinates&oldid=7658"