Triangularity: Difference between revisions
Jump to navigation
Jump to search
No edit summary |
m Add simple illustration of shift, elongation, triangularity, and squareness |
||
| (5 intermediate revisions by 2 users not shown) | |||
| Line 1: | Line 1: | ||
[[File:Geometry.png|400px|thumb|right|Sketch of tokamak geometry, including separatrix]] | [[File:Geometry.png|400px|thumb|right|Sketch of tokamak geometry, including separatrix]] | ||
[[File:Cross_section_1shift_2elong_3triang_4square.png|400px|thumb|right|Illustration of the m=1,2,3, and 4 perturbations to a tokamak plasma cross section. Triangularity is the m=3 perturbation.]] | |||
The triangularity refers to the shape of the poloidal cross section of the Last Closed [[Flux surface]] (LCFS) or [[separatrix]] of a [[tokamak]]. | The triangularity refers to the shape of the poloidal cross section of the Last Closed [[Flux surface]] (LCFS) or [[separatrix]] of a [[tokamak]]. | ||
Assuming: | Assuming<ref>T.C. Luce, [[doi:10.1088/0741-3335/55/9/095009|Plasma Phys. Control. Fusion '''55''' (2013) 095009 ]]</ref>: | ||
* ''R<sub> | * ''R<sub>max</sub>'' is the maximum value of ''R'' along the LCFS or separatrix. | ||
* ''a'' is the minor radius of the plasma, defined as | * ''R<sub>min</sub>'' is the minimum value of ''R'' along the LCFS or separatrix. | ||
* ''R<sub>upper</sub>'' is the major radius of the highest vertical point of the LCFS. | * ''R<sub>geo</sub>'' is the geometric major radius, defined as ''(R<sub>max</sub> + R<sub>min</sub>)/2''. | ||
* ''R<sub>lower</sub>'' is the major radius of the lowest vertical point of the LCFS. | * ''a'' is the minor radius of the plasma, defined as ''(R<sub>max</sub> - R<sub>min</sub>)/2''. | ||
* ''R<sub>upper</sub>'' is the major radius of the highest vertical point of the LCFS or separatrix. | |||
* ''R<sub>lower</sub>'' is the major radius of the lowest vertical point of the LCFS or separatrix. | |||
The upper triangularity is then defined as follows: | The upper triangularity is then defined as follows: | ||
:<math> \delta_{upper} = ( | :<math> \delta_{upper} = (R_{geo}-R_{upper})/a</math> | ||
and similar for δ<sub>lower</sub>. | and similar for δ<sub>lower</sub>. | ||
The overall triangularity is defined as the mean of δ<sub>upper</sub> and δ<sub>lower</sub>. | The overall triangularity is defined as the mean of δ<sub>upper</sub> and δ<sub>lower</sub>. | ||
Triangularity, especially the triangularity opposite the dominant X-point (so upper triangularity for a lower null plasma), influences the stability and character of the [[pedestal]] and [[Edge Localized Modes|ELMs]].<ref>[[doi:10.1088/0741-3335/42/5A/319|T.H. Osborne, et al., Plasma Phys. Control. Fusion '''42''' (2000) A175]]</ref> | |||
Some devices (TCV and DIII-D) can form plasma cross sections with negative triangularity (the X-points are pushed to larger <math>R</math> than the center of the plasma), which makes H-mode difficult or impossible to access but improves performance of the L-mode.<ref>[[doi:10.1088/1741-4326/abdb95|W. Han, et al., Nucl. Fusion '''61''' (2021) 034003]]</ref> | |||
== See also == | == See also == | ||
| Line 16: | Line 23: | ||
* [[Toroidal coordinates]] | * [[Toroidal coordinates]] | ||
* [[Effective plasma radius]] | * [[Effective plasma radius]] | ||
== References == | |||
<references /> | |||
Latest revision as of 22:50, 30 March 2023
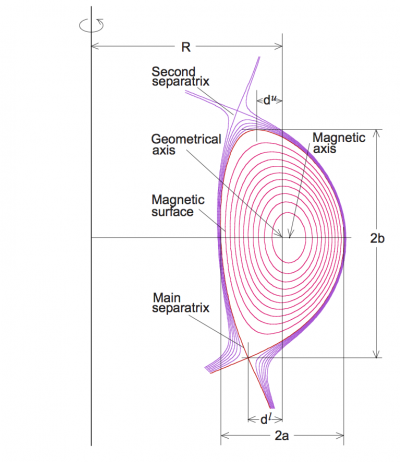

The triangularity refers to the shape of the poloidal cross section of the Last Closed Flux surface (LCFS) or separatrix of a tokamak. Assuming[1]:
- Rmax is the maximum value of R along the LCFS or separatrix.
- Rmin is the minimum value of R along the LCFS or separatrix.
- Rgeo is the geometric major radius, defined as (Rmax + Rmin)/2.
- a is the minor radius of the plasma, defined as (Rmax - Rmin)/2.
- Rupper is the major radius of the highest vertical point of the LCFS or separatrix.
- Rlower is the major radius of the lowest vertical point of the LCFS or separatrix.
The upper triangularity is then defined as follows:
and similar for δlower. The overall triangularity is defined as the mean of δupper and δlower.
Triangularity, especially the triangularity opposite the dominant X-point (so upper triangularity for a lower null plasma), influences the stability and character of the pedestal and ELMs.[2]
Some devices (TCV and DIII-D) can form plasma cross sections with negative triangularity (the X-points are pushed to larger than the center of the plasma), which makes H-mode difficult or impossible to access but improves performance of the L-mode.[3]