Flux surface: Difference between revisions
No edit summary |
No edit summary |
||
| (21 intermediate revisions by the same user not shown) | |||
| Line 3: | Line 3: | ||
:<math>\vec B \cdot \vec n = 0</math> | :<math>\vec B \cdot \vec n = 0</math> | ||
everywhere. | everywhere on ''S''. | ||
In other words, the magnetic field does not ''cross'' the surface ''S'' anywhere, i.e., the magnetic flux traversing ''S'' is zero. | |||
It is then possible to define a scalar ''flux function'' (''f'') such that its value is constant on the surface ''S'', and | |||
:<math>\vec B \cdot \vec \nabla f = 0</math> | :<math>\vec B \cdot \vec \nabla f = 0</math> | ||
In three dimensions, the only closed flux surface corresponding to a ''non-vanishing'' vector field is a topological toroid. | In three dimensions, the only closed flux surface corresponding to a ''non-vanishing'' vector field is a topological toroid. | ||
<ref>The Poincaré-Hopf Theorem.</ref> | <ref>[[:Wikipedia:Hairy_ball_theorem|The Poincaré-Hopf Theorem]].</ref> | ||
This fact lies at the basis of the design of magnetic confinement devices. | This fact lies at the basis of the design of magnetic confinement devices. | ||
Assuming the flux surfaces have this toroidal topology, the function ''f'' defines a set of ''nested'' surfaces, so it makes sense to use this function to label the flux surfaces, i.e., ''f'' may be used as a "radial" coordinate. Each toroidal surface ''f'' encloses a volume ''V(f)''. | |||
The surface corresponding to an infinitesimal volume ''V'' is essentially a line that corresponds to | |||
the ''toroidal axis'' (called ''magnetic axis'' when ''B'' is a magnetic field). | |||
The flux ''F'' through an arbitrary surface ''S'' is given by | |||
:<math>F = \int_S{\vec B \cdot \vec n dS}</math> | |||
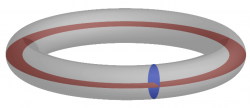
[[File:Flux_definition.png|250px|thumb|right|Diagram showing the surfaces defining the poloidal (red) and toroidal (blue) flux]] | |||
When ''B'' is a magnetic field with toroidal nested flux surfaces, two magnetic fluxes can be defined from two corresponding surfaces. | |||
<ref>R.D. Hazeltine, J.D. Meiss, ''Plasma Confinement'', Courier Dover Publications (2003) {{ISBN|0486432424}}</ref> | |||
The poloidal flux is defined by | |||
:<math>\psi = \int_{S_p}{\vec B \cdot \vec n dS}</math> | |||
where ''S<sub>p</sub>'' is a ring-shaped ribbon stretched between the magnetic axis and the flux surface ''f''. | |||
(Complementarily, ''S<sub>p</sub>'' can be taken to be a surface spanning the central hole of the torus.<ref>[http://link.aps.org/doi/10.1103/RevModPhys.76.1071 A.H. Boozer, ''Physics of magnetically confined plasmas'', Rev. Mod. Phys. '''76''' (2005) 1071 - 1141]</ref>) | |||
Likewise, the toroidal flux is defined by | |||
:<math>\phi = \int_{S_t}{\vec B \cdot \vec n dS}</math> | |||
where ''S<sub>t</sub>'' is a poloidal section of the flux surface. | |||
It is natural to use ψ or φ to label the flux surfaces instead of the unphysical label ''f''. | |||
== See also == | |||
* [[MHD equilibrium]] | |||
* [[Toroidal coordinates]] | |||
* [[Flux coordinates]] | |||
* [[Rotational transform]] | |||
* [[Magnetic shear]] | |||
* [[Effective plasma radius]] | |||
* [[Separatrix]] | |||
* [[Flux tube]] | |||
== References == | == References == | ||
<references /> | <references /> | ||
Latest revision as of 11:38, 26 January 2023
A given smooth surface S with normal n is a flux surface of a smooth vector field B when
everywhere on S. In other words, the magnetic field does not cross the surface S anywhere, i.e., the magnetic flux traversing S is zero. It is then possible to define a scalar flux function (f) such that its value is constant on the surface S, and
In three dimensions, the only closed flux surface corresponding to a non-vanishing vector field is a topological toroid. [1] This fact lies at the basis of the design of magnetic confinement devices.
Assuming the flux surfaces have this toroidal topology, the function f defines a set of nested surfaces, so it makes sense to use this function to label the flux surfaces, i.e., f may be used as a "radial" coordinate. Each toroidal surface f encloses a volume V(f). The surface corresponding to an infinitesimal volume V is essentially a line that corresponds to the toroidal axis (called magnetic axis when B is a magnetic field).
The flux F through an arbitrary surface S is given by
When B is a magnetic field with toroidal nested flux surfaces, two magnetic fluxes can be defined from two corresponding surfaces. [2] The poloidal flux is defined by
where Sp is a ring-shaped ribbon stretched between the magnetic axis and the flux surface f. (Complementarily, Sp can be taken to be a surface spanning the central hole of the torus.[3]) Likewise, the toroidal flux is defined by
where St is a poloidal section of the flux surface. It is natural to use ψ or φ to label the flux surfaces instead of the unphysical label f.
See also
- MHD equilibrium
- Toroidal coordinates
- Flux coordinates
- Rotational transform
- Magnetic shear
- Effective plasma radius
- Separatrix
- Flux tube
References
- ↑ The Poincaré-Hopf Theorem.
- ↑ R.D. Hazeltine, J.D. Meiss, Plasma Confinement, Courier Dover Publications (2003) ISBN 0486432424
- ↑ A.H. Boozer, Physics of magnetically confined plasmas, Rev. Mod. Phys. 76 (2005) 1071 - 1141