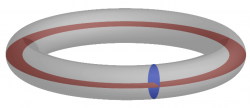
Flux surface
A given smooth surface S with normal n is a flux surface of a smooth vector field B when
everywhere. Defining a scalar flux function (f) such that its value is constant on the surface S, this can be rewritten
In three dimensions, the only closed flux surface corresponding to a non-vanishing vector field is a topological toroid. [1] This fact lies at the basis of the design of magnetic confinement devices.
Assuming the flux surfaces have this toroidal topology, the function f defines a set of nested surfaces, so it makes sense to use this function to label the flux surfaces, i.e., f may be used as a "radial" coordinate. Each toroidal surface f encloses a volume V(f). The surface corresponding to an infinitesimal volume V is essentially a line that corresponds to the toroidal axis (called magnetic axis when B is a magnetic field).
The flux F through an arbitrary surface S is given by
When B is a magnetic field with toroidal nested flux surfaces, two magnetic fluxes can be defined from two corresponding surfaces. [2] The poloidal flux is defined by
where Sp is a ring-shaped ribbon stretched between the magnetic axis and the flux surface f, and the toroidal flux by
where St is a poloidal section of the flux surface. It is natural to use ψ or φ to label the flux surfaces instead of the unphysical label f.
See also
References
- ↑ The Poincaré-Hopf Theorem.
- ↑ R.D. Hazeltine, J.D. Meiss, Plasma Confinement, Courier Dover Publications (2003) ISBN 0486432424